Many heads are better than one: 9 colours activity

Fantastic example of collaboration and the amazing people I work with occurred today. The school year has started and after two days of presentations down on the Peninsula I am back in the office today, preparing for a full week of school visits next week.

I’m really exciting about working with the teachers and year 9 students at Dromana College next week, over two afternoons. I want to really make an impression with the students, with a growth mindset presentation, followed by a couple of challenging and fun activities.

The presentation I plan on showing comes from the wonderful Jo Boaler’s website https://www.youcubed.org/. She is so inspiring and I talk about her work in all my schools. The presentation is about maths, believing in yourself, the importance of mistakes and the beauty of maths. Now I needed an activity to encourage and enable collaboration.

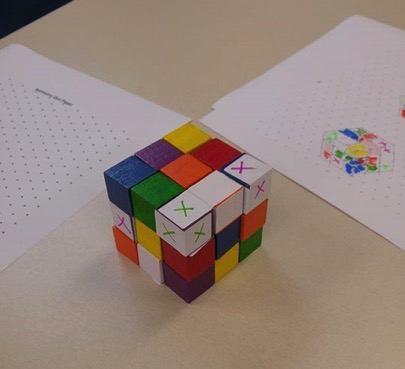
Going to nrich I found ‘9 colours’ which I believed looked thought-provoking so I asked my colleagues if they were interested in solving it collaboratively, to help determine how successful it could be. Being in a room full of mathematicians and maths teachers, they jumped at it and the conversation flowed.

Initially, as always happens, someone jumped in and started assembling the first level, which, let’s be honest, isn’t particularly difficult requiring 9 cubes and 9 different colours. The second level was assembled just as quickly before someone called a halt to proceedings and insisted we started thinking more carefully about the placement of cubes. This led to discussion around which colour would sit in the very centre and where the two other cubes of that colour needed to be placed.

We would have made it a great deal easier on ourselves if we had started assemble by laying out the bottom level first and building up, but we started from one side and moved across. This resulted in the need to physically hold cubes in place as we set about placing all cubes of each colour, rather than working level by level. This would have saved the mistake we made when we checked the base; misplaced colours. Correcting this led to further discussion around how many faces of each colour were visible in the completed cube.

Having completed the large cube, discussion turned to how we could develop and extend the activity. Depicting the completed cube on isometric paper seemed to next logical step. 3-D drawings are often difficult for students, something that frequently shows up in NAPLAN results at all levels. Enabling students to first draw 3-D objects in 2-D, and then recreate from 2-D back into 3-D, develops their spatial awareness. Redrawing the large cube after a specific colour has been removed develops this even more.

As we worked through the task, more and more ideas emerged on how to take an engaging activity and turn it in a rich task, suitable as a multi-ability, multi-age investigation. Through collaboration and discussion we were able to relate it to the Australian Curriculum at 6 different year levels, extending it in many ways:

• Draw it on isometric paper from different perspectives.
• How many drawings do you need to show all faces?
• Is there more than one way to place each small cube?
• Is yours the same as another group’s cube?
• If you gave another group a drawing could they reconstruct your design?
  • What is the minimum amount of information you need to give them?
• Is there a pattern to the placement of your cubes?
• Remove a particular colour (3 blocks), then draw the design with missing blocks.
• What is the surface area and the volume?
• How does the surface area and volume change when you remove one colour?
• Can you predict the colour of the middle block (the one not visible)?
• Is there symmetry in your design?
• Can you determine the total number of combinations?
• What can you determine about the number of faces of each colour you will see in the completed cube?
• Can you explain this using numbers/algebra?
• How many colours would you need for a 4 by 4 by 4 cube?
• How many cubes in a 4 by 4 by 4 cube?
• Do the same rules and patterns exist in a 4 by 4 by 4 cube?
• Is there a point at which the task is impossible? (5 x 5 x 5; 6 x 6 x 6; 7 x 7 x 7…)