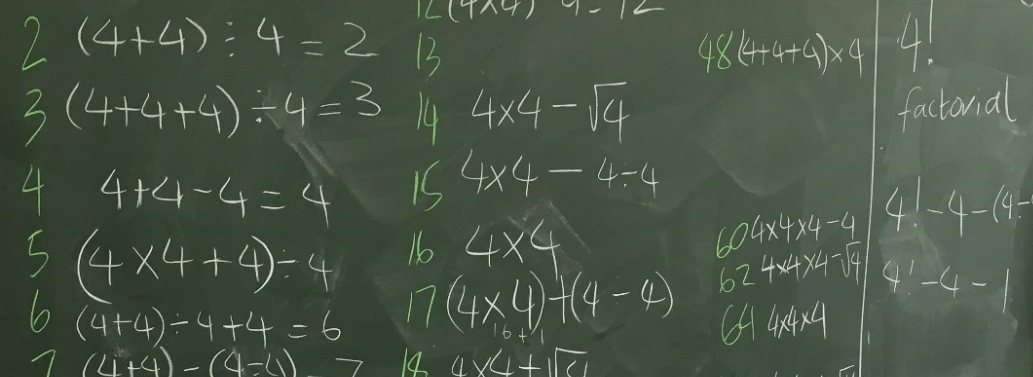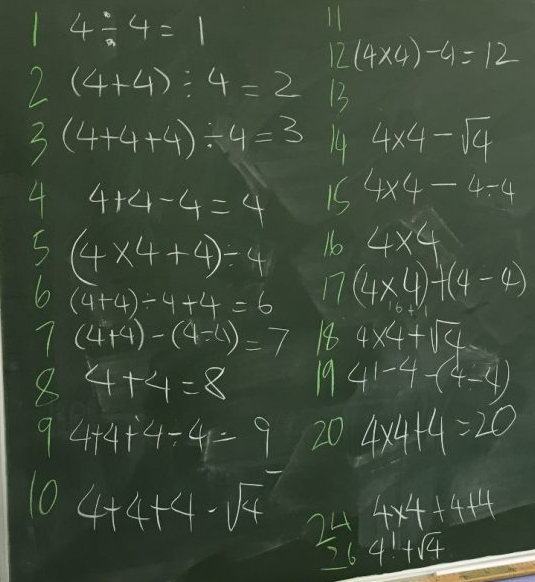Why?
This lesson has been designed for students in Upper Primary. It builds on the problem “Four Goodness Sake” found on the NRICH website (https://nrich.maths.org/1081). It is aimed at helping students develop an increased understanding into the order of operations and how the use of brackets in an equation can modify the solution.
What?
The lesson activity requires students to use up to four fours and any operation to make the numbers from 0 to 100. For example, 4 + 4 + 4 + 4 = 16.
Initially, students will use the more familiar operations, +, −, ×, ÷, along with brackets to create their equations. For example, (4 × 4 + 4) ÷ 4 = 5. Gradually, finding solutions for the remaining numbers in the list becomes more challenging. Here, it is a good opportunity to introduce some other operations to the students, including √4 = 2 and 4! = 24. In my experience, at least one student in the class will be familiar with the square root operation √n, which will make it easier to introduce. The other operation n!, known as factorial, will be less familiar to students.
n! is the product of all positive integers less than or equal to n; for example, 4! = 4 × 3 × 2 × 1 = 24, or 3! = 3 × 2 × 1 = 6
With the introduction of these two less familiar operations, the number of solutions that students can find to the initial problem increases.
How?
Recently, in a 5/6 class in NSW the students were quick to understand the initial problem. After naming some of the more obvious solutions, the challenge soon came to find solutions for the numbers from 1 to 10. We decided to use the large classroom blackboard to record the solutions as they were suggested. On the side of the board a “working out” space was included so problems where the “order of operations” was a factor could be tested before being added to the main list.
Although several solutions larger than 10 were also discovered, the number 10 itself became a bit of a sticking point. It was here that I prompted students to think of using different operations. One student suggested , but soon realised that this would not be allowed as it involved using a number other than 4. Another student said that the opposite to “squared” was “square root” and soon a new operation was available to the students. This “new” knowledge was then used by students to provide multiple solutions for the number 10, including:
4 + 4 + 4 – √4
4 + 4 + √4
After another five to ten minutes of working, when the sharing of possible solutions had again slowed down I introduced the factorial operation (. Not only did this help students find solutions to some different numbers, it also helped them to develop less complicated solutions to some of previous numbers. For example:
4! ÷ 4 = 6
With this new information in place, over the course of one hour, the class of students were able to develop solutions to more than 30 numbers. As the lesson was finishing, students were still eagerly sharing potential solutions.
Dig deeper
For more information, the attached lesson plan shows the relevant links to the Australian Curriculum and highlights the related content and outcomes as listed in the NSW Mathematics K-10 syllabus.